10.2: Phenetics
( \newcommand{\kernel}{\mathrm{null}\,}\)
The other way of making classification is even more mathematical. This is phenetics based on multivariate methods of data analysis. One of its methods is cluster analysis which is described below.
- Contrary to cladistics, phenetics considers characters as all equal and does not employ any evolutionary assumptions.
- We need to decide which taxa we will need, assess their descriptions, extract characters—all these is similar to cladistics (see above).
- Character polarization is not needed, character codes may be specified more or less arbitrarily, and there is no need for outgroup.
- Character table could be the same as in previous example (again, see above).
- Then, we will need to create the square matrix (or table) of similarity:
Alphaceae Betaceae Gammaceae Alphaceae 1 Betaceae 0.75 1 Gammaceae 0.25 0 1 Every cell of this matrix contains a value of similarity K: K=number of matching charactersnumber of all characters
Please note that there are many more relevant coefficients of similarity but they are out of our scope.
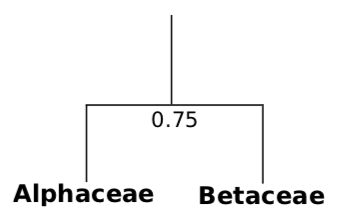
- Then we need to make the dendrogram which is a tree-like structure. Tradi- tionally, dendrogram is built from bottom to top, from more similar to less similar groups. For example, we may start from connecting the closest taxa, Alphaceae and Betaceae:
7. Then we need to attach other taxa which are closest to previous group:
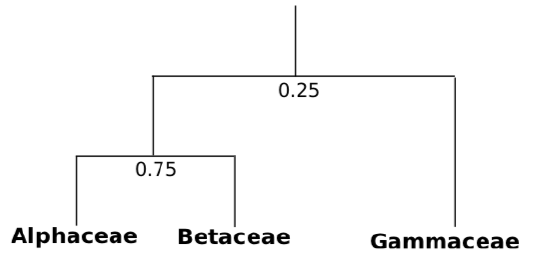
Sometimes, when we have multiple taxa, we end up with several independent groups (clusters). In that case, different clusters could be connected on the base of average similarity.
8. Betaceae and Alphaceae are closer, so we can unite them in one order:
Order Alphales
1. Family Alphaceae 2. Family Betaceae
Order Gammales
1. Family Gammaceae


