14.4: Concentration Control and Elasticity Coefficients

Search Fundamentals of Biochemistry

Learning Goals (ChatGPT o3-mini)

1. Define Concentration Control Coefficients:

   • Explain the concept of a concentration control coefficient (\(C_{E_{i}}^{S}\)) as a measure of how changes in an enzyme’s activity affect the concentration of a system metabolite.
   • Interpret the equation (\(C_{E_i}^S=\frac{\frac{\partial S_i}{S_i}}{\frac{\partial u_i}{u_i}}=\frac{\partial \ln S_i}{\partial \ln u_i}=\frac{\partial S_i}{\partial u_i} \frac{u_i}{S_i}
     \)), emphasizing its role as a system-level property.

2. Understand the Summation Theorem for Concentration Coefficients:

   • Demonstrate why the sum of all individual concentration control coefficients in a pathway equals zero, 
     \(\sum_{i=1}^n C_{E i}^S=0
     \)
      
   • Discuss the implications of this theorem for the redistribution of control within a metabolic network.

3. Interpret the Impact of Enzyme Activity Changes on Metabolite Concentrations:

   • Analyze how a decrease in enzyme activity (e.g., due to a mutation) can lead to compensatory changes in substrate concentrations, using the example of a low [S] scenario where [S] must increase to maintain constant flux.

4. Define and Calculate Elasticity Coefficients:

   • Describe the elasticity coefficient (\(\varepsilon_{S}^{v}\))  as a local property that quantifies how sensitive an enzyme’s rate (v) is to changes in substrate concentration (S).
   • Explain the elasticity equation (\(\varepsilon_S^v=\frac{\frac{\partial v}{v}}{\frac{\partial S}{S}}=\frac{\partial \ln v}{\partial \ln S}=\frac{\partial v}{\partial S} \frac{S}{v}\)),

5. Relate Elasticity Coefficients to Enzyme Kinetics:

   • Explain how elasticity coefficients can be determined from the slope of a velocity vs. substrate concentration curve at a specific tangent point and why they must be evaluated under in vivo steady-state conditions.
   • Differentiate between elasticities for substrates, products, and other modifiers, noting that values can be positive or negative depending on whether the species increases or decreases the reaction rate.

6. Connect System-Level and Local Control Parameters:

   • Explain the rationale behind linking global system control coefficients (like flux and concentration control coefficients) to local properties (elasticity coefficients) via the Connectivity Theorem: (\(\Sigma_{i=1}^n C_{v i}^J e_s^{v i}=0\))
   • Analyze how this theorem integrates multiple enzyme influences at branch points and complex network nodes.

7. Apply Computational Tools for Parameter Analysis:

   • Use software such as COPASI to analyze and interpret tables of elasticity coefficients and concentration control coefficients from experimental data.
   • Evaluate how changes in kinetic parameters (K_m, V_m, K_ix) affect system flux and metabolite concentrations, reinforcing the connection between theory and practical measurements.

8. Critically Assess the Role of Control Coefficients in Metabolic Regulation:

   • Evaluate how concentration control coefficients differ from flux control coefficients in terms of their magnitude, range (negative to positive for concentration coefficients vs. 0 to 1 for flux coefficients), and overall impact on pathway regulation.
   • Discuss real-world examples and experimental findings that illustrate the importance of these coefficients in maintaining metabolic homeostasis.

These learning goals are designed to guide students through both the theoretical derivations and practical implications of concentration control and elasticity coefficients, equipping them with a deeper understanding of how enzyme kinetics shape the behavior of entire metabolic systems.

Concentration Control Coefficients

The Concentration control coefficient (\(C_{E_{i}}^{S}\)), a global property of the system, gives the relative fractional change in metabolite concentration \(S_j (dS_j/S_j)\), where \(S_j\) is the concentration of any metabolite in the system and as such is a system variable, with fractional change in concentration or activity of enzyme \(E_i (du_i/u_i)\). Similar equations as section 15.3 apply.

\begin{equation}
C_{E_i}^S=\frac{\frac{\partial S_i}{S_i}}{\frac{\partial u_i}{u_i}}=\frac{\partial \ln S_i}{\partial \ln u_i}=\frac{\partial S_i}{\partial u_i} \frac{u_i}{S_i}
\end{equation}

It can be shown that the sum of all of the individual \(C^S_{E_i} = 0\) (another summation theorem) is not 1, as in the case of flux control coefficients. This again would make sense in the steady state. Flux coefficients usually vary from 0 to 1, but concentration coefficients can vary from negative to positive and small to large.

\begin{equation}
\sum_{i=1}^n C_{E i}^S=0
\end{equation}

A simple example shows that the concentration control coefficients can have large values. For a given enzyme, at low [S], for example, when [S] << Km,

\begin{equation}
v=\frac{V_m S}{K_M+S}=\frac{V_m S}{K_M}=\frac{k_{c a t} E_{t o t} S}{K_M} \text { when } S \ll K m
\end{equation}

If the enzyme had only 0.1x of its normal activity (due to a mutation, for example), then to maintain constant flux, the [S] would have to increase 10-fold.

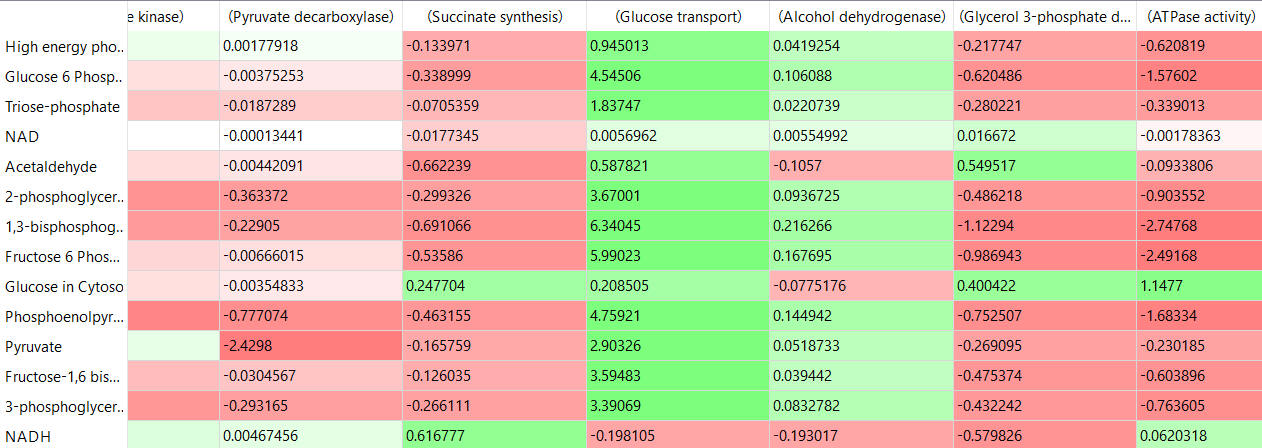
The tables below show the C^S_Ei values for incremental changes in the substrate (1%).

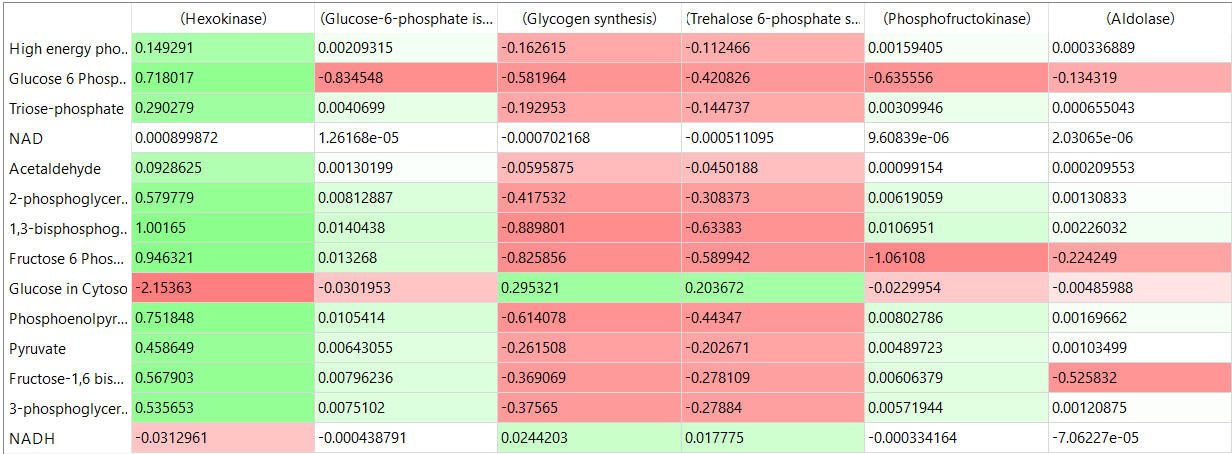
table, part 2:

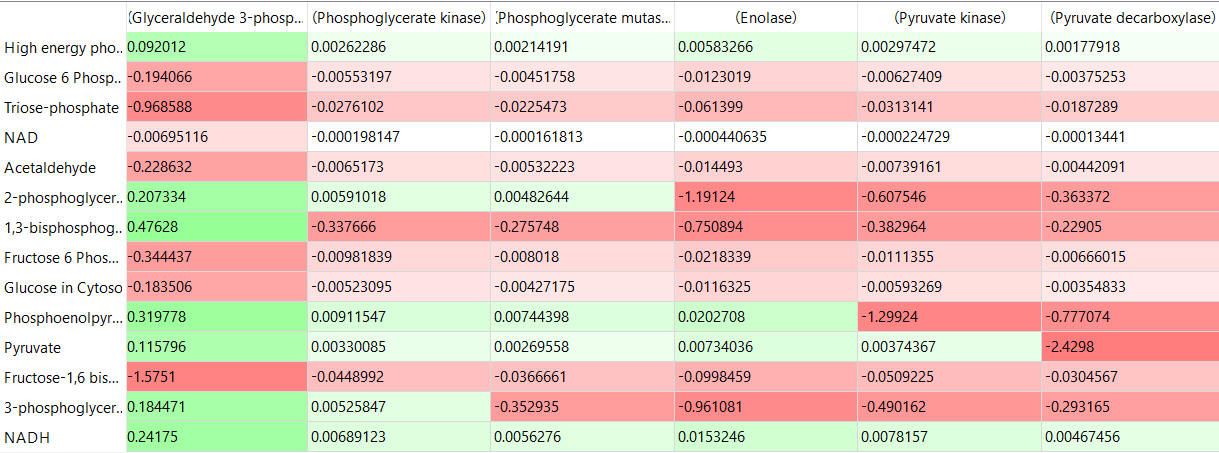
table, part 3

Elasticity Coefficient

The Elasticity Coefficient, (\(\varepsilon_{S}^{v}\)), in contrast to the flux and concentration control coefficients, which are properties of the system, is a local property and can be measured using isolated enzymes and substrates. It makes sense that some kinetic property of the isolated enzyme would affect its propensity to affect system flux. The elasticity coefficient gives a measure of how much a substrate \(S\) (or other substance) can change the reaction rate (\(v\)) of an isolated enzyme. (Note that we use \(v\) and not flux \(J\), which describes a system property.) Hence

\begin{equation}
\varepsilon_S^v=\frac{\frac{\partial v}{v}}{\frac{\partial S}{S}}=\frac{\partial \ln v}{\partial \ln S}=\frac{\partial v}{\partial S} \frac{S}{v}
\end{equation}

Price Elasticity

The term elasticity is also used in economics and is especially useful when inflation is high. If the price of your favorite product, such as a Starbucks Latte, goes up, consumers might buy them less frequently or buy another cheaper latte from a competitor. If a small rise in price for a Starbucks latte leads to a large drop in demand, the Starbucks latte is characterized by a high elasticity. However, if people don't change their latte buying behavior when there is a big price rise on the latte, the product is inelastic. If you are the CEO of a company, it is good to know the elasticity of your products to maximize your profits.

Hence, the elasticity coefficient can be determined using basic enzyme kinetics of the isolated enzyme. Note that the coefficient at each \(S\) concentration is the slope of the v vs S curve multiplied by the \(S/v\) at that tangent point. The elasticity coefficient must be evaluated at the same enzyme and substrate concentration as in vivo in the steady state. Velocity (\(v\)), not flux, is used. In the above case, \(S\) is the substrate, but it could be a product or modifier. There is a different elasticity coefficient for each parameter. There is no summation theory for elasticities. Values can be positive for species that increase the velocity or negative for those that decrease it. Hence there can be multiple elasticities.

The tables below show the elasticity coefficients (relative or scaled) for yeast glycolysis determined using COPASI.

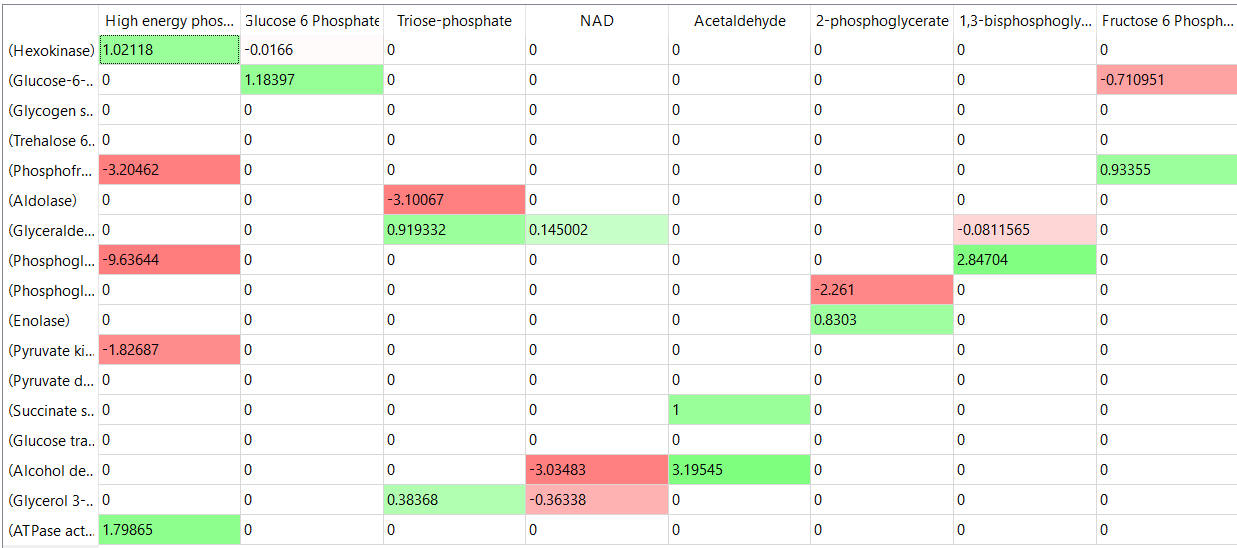
table, part 2

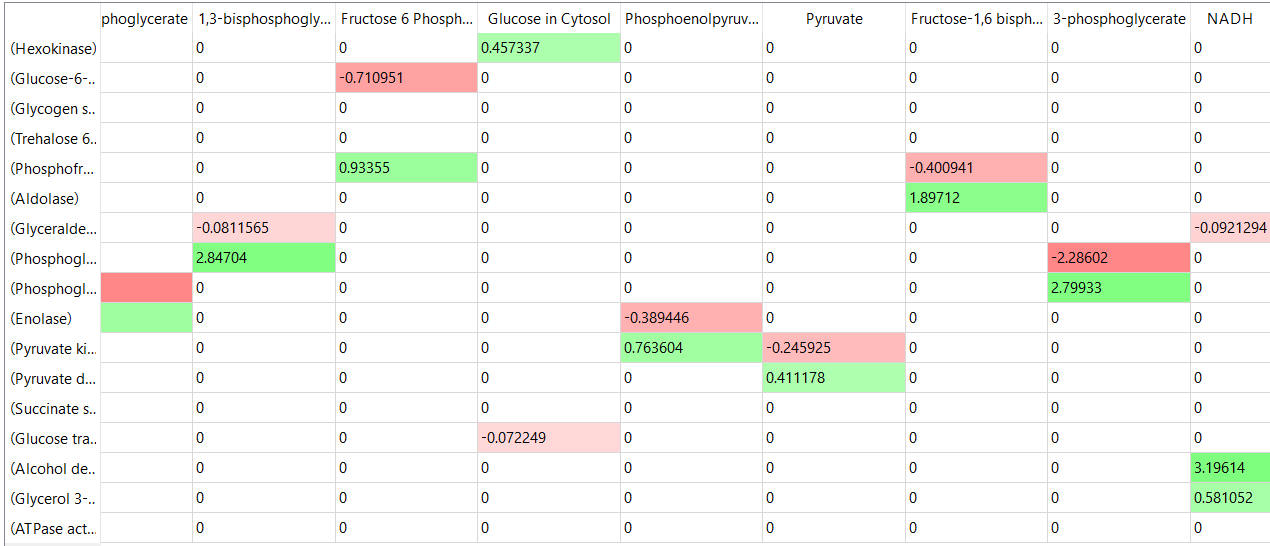
Things to note:

• the columns show substrates not enzymes;
• green cells (with positive elasticities) are generally substrates for their target enzymes (for example, glucose-6-phosphate for phosphoglucomutase);

Other "generic" sensitivities can be determined as well. For example, incremental changes in \(K_m\), \(V_m\), or \(K_{ix}\) for specific enzymes could affect fluxes in a pathway.

A link between system control coefficients and local coefficients:

You would think there should be some relationship between a system variable such as the flux control coefficient and a local variable such as the elasticity coefficient. There is, and it is expressed by the Connectivity Theorem (below). If a substrate \(S\) is acted upon by many different enzymes (\(i …… n\)), which is very likely, especially for branch points in metabolic pathways, then it can be shown that

\begin{equation}
\sum_{i=1}^n C_{v i}^J e_s^{v i}=0
\end{equation}

Summary

This chapter delves into two key concepts in metabolic control analysis: concentration control coefficients and elasticity coefficients. It begins by defining the concentration control coefficient (\(C_{E_{i}}^{S}\)) as a system-level parameter that quantifies the relative change in a metabolite’s concentration in response to a fractional change in the activity or concentration of an enzyme. Mathematically, it is expressed as

\(C_{E_i}^S=\frac{\frac{\partial S_i}{S_i}}{\frac{\partial u_i}{u_i}}=\frac{\partial \ln S_i}{\partial \ln u_i}=\frac{\partial S_i}{\partial u_i} \frac{u_i}{S_i}
\),

which highlights its dependence on both local enzyme properties and global metabolite levels. A critical insight is provided by the summation theorem for concentration coefficients, which states that the sum of all individual concentration control coefficients within a pathway equals zero (\(\sum_{i=1}^n C_{E i}^S=0
\)). This result underscores the idea that changes in enzyme activities are balanced across the system, reflecting the interconnected nature of metabolic networks.

The chapter then introduces the elasticity coefficient, (\(\varepsilon_{S}^{v}\)), a local property that measures how sensitively an enzyme’s reaction rate (v) responds to changes in the concentration of a substrate or modifier. Defined as

\(\varepsilon_S^v=\frac{\frac{\partial v}{v}}{\frac{\partial S}{S}}=\frac{\partial \ln v}{\partial \ln S}=\frac{\partial v}{\partial S} \frac{S}{v}\),

the elasticity coefficient is determined by the slope of the vv versus SS curve at a given point and must be evaluated under conditions that mimic the in vivo steady state. Unlike system-level control coefficients, elasticity coefficients can have both positive and negative values, reflecting whether a substrate acts to enhance or inhibit enzyme activity.

An important connection between local and global properties is established through the Connectivity Theorem, which links flux control coefficients and elasticity coefficients by the relation

(\(\Sigma_{i=1}^n C_{v i}^J e_s^{v i}=0\))

This theorem integrates the local responsiveness of individual enzymes with the overall control exerted on the system flux, emphasizing that the effects of enzyme modifications are distributed throughout the network.

Overall, the chapter provides a comprehensive framework for understanding how local enzyme kinetics (as measured by elasticity coefficients) relate to and influence the global behavior of metabolic systems (as characterized by concentration control coefficients). By combining theoretical derivations with computational analyses, students gain a nuanced perspective on how changes at the molecular level can impact whole-pathway dynamics, offering valuable insights into metabolic regulation and system robustness.