1.2: Genes are the Units of Heredity: Mendel's Laws
- Page ID
- 303
Mendel's First Law: Alleles segregate equally
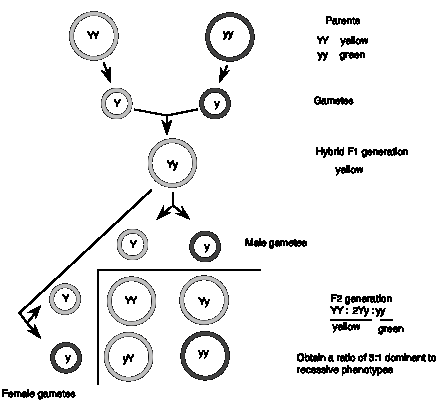
The original experiments by Gregor Mendel involved phenotypic traits (physical, observable characteristics) controlled by single genes. The first one we'll consider is seed color, which can be yellow or green. The dominant allele, denoted Y, generates yellow peas in either the homozygous (YY) or heterozygous (Yy) state, whereas the recessive allele, denoted y, generates green peas only in the homozygous state (yy). (In plants and flies, the dominant allele is denoted by a capitalized abbreviation and the recessive allele is denoted by a lower case abbreviation.) In a cross between two parents, one homozygous for the dominant allele (YY) and the other homozygous for the recessive allele (yy), Mendel showed that the F1 progeny were all yellow, i.e. they had had the same phenotype as the parent with the dominant allele. The recessive allele was not contributing to the phenotype.
Had it been lost during the cross? No, when the F1 is crossed with itself, both parental phenotypes were seen in the F2 progeny. The effect of the recessive allele reappeared in the second cross, showing that it was still present in the F1 hybrids, but was having no effect. In the F2 progeny, the dominant phenotype (yellow) was observed in 75% of the progeny and the recessive (green) appeared in only 25% of the progeny.
Note that discrete phenotypes were obtained (yellow or green), not a continuum of phenotypes. The genes are behaving as units, not as some continuous function.
The results can be explained by hypothesizing that each parent has two copies of the gene (i.e., two alleles) that segregate equally, one per gamete. Since they are homozygous, each parent can form only type of gamete (Y or y,respectively). When the gametes join in the zygotes of the F1 generation, each individual receives one dominant allele and one recessive allele (Yy), and thus all of the F1 generation shows the dominant phenotype (e.g. yellow peas). This is the uniform phenotype observed for the F1 generation.
The two alleles did not alter one another when present together in the F1 generation, because when F1 is crossed with F1, the two parental phenotypes are obtained in the F2 generation.
The ratio of 3:1 dominant: recessive observed in the F2 is expected for the equal segregation of the alleles from the F1(Y and y) and their random rejoining in the zygotes of the F2, producing the genotypes 1 YY, 2 Yy, and 1 yy. Again the genes are behaving as discrete units. These precise mathematical ratios (3:1 for phenotypes in this cross, or 1:2:1 for the genotype) provide the evidence that genes, units of heredity, are determining the phenotypes observed.
Not all loci show the property of complete dominance illustrated by the Y locus in peas. Sometimes partial dominance is observed, in which an intermediate phenotype seen in a heterozygote. An example is the pink color of snapdragons obtained when white and red are crossed. However, the parental phenotypes reappear in the F2 generation, showing that the alleles were not altered in the heterozygote. In this case, gene dosage is important in determining the phenotype; two wild-type alleles produce a red flower, but only one wild-type allele produces a pink flower. Sometimes co‑dominance is observed, in which both alleles contribute equally to the phenotype. An example is the ABO blood group locus. Heterozygotes have both the A and B form of the glycoprotein that is encoded by the different alleles of the gene.
Mendel's Second Law: Different Genes Assort Independently
Now that we have some understanding of the behavior of the different alleles of a single gene, let's consider how two different genes behave during a cross. Do they tend to stay together, or do they assort independently?
Mendel examined two different traits, seed color (as described in the previous section) and seed shape. Two alleles at the locus controlling seed shape were studied, the dominant round (R) and recessive wrinkled (r) alleles. Mendel crossed one parent that was homozygous for the dominant alleles of these two different genes (round yellow RRYY) with another parent that was homozygous for the recessive alleles of those two genes (wrinkled green rryy) (see Figure 1.2).
Re-stating the basic question, do the alleles at each locus always stay together (i.e. round with yellow, wrinkled with green) or do they appear in new combinations in the progeny? As expected from the 1st law, the F1 generation shows a uniform round yellow phenotype, since one dominant and one recessive allele was inherited from the parents. When the F2 progeny are obtained by crossing the F1 generation, the parental phenotypes reappear (as expected from the first law), but two nonparental phenotypes also appear that differ from the parents: wrinkled yellow and round green!
The results can be explained by the alleles of each different gene assorting into gametes independently. For example, in the gametes from the F1 generation, R can assort with Yor y, and r can assort with Y or y, so that four types of gametes form: RY, Ry, rY, and ry. These can rejoin randomly with other gametes from the F1 generation, producing the results in the grid shown in Figure 1.2. The alternative, that R always assorted with Y, etc. was not observed.
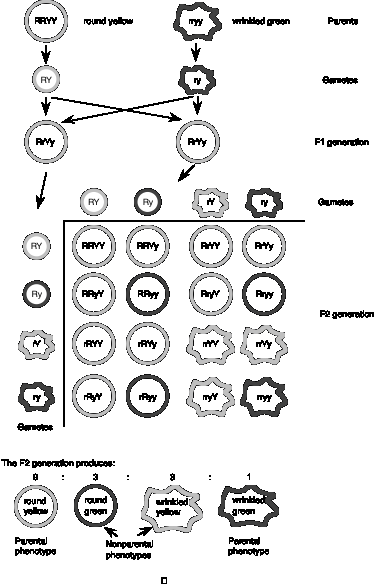
Again, the genes are behaving as units, and the gene for one trait (e.g. color) does not affect a gene for another trait (e.g. shape). Further breeding shows that many nonparental genotypes are present, some of which give a parental phenotype (e.g. RrYy). These results are obtained for genes that are not linked on chromosomes. Linkage can lead to deviations from these expected ratios in a mating, and this can be used to map the locations of genes on chromosomes, as discussed in the next section.


