ATP*#
( \newcommand{\kernel}{\mathrm{null}\,}\)
ATP
An important chemical compound is adenosine triphospate (ATP). The main cellular role of ATP is as a "short-term" energy transfer device for the cell. The hydrolysis reactions that liberate one or more of ATP's phosphates are exergonic and many, many cellular proteins have evolved to interact with ATP in ways that help facilitate the transfer of energy from hydrolysis to myriad other cellular functions. In this way, ATP is often called the “energy currency” of the cell: it has reasonably fixed values of energy to transfer to or from itself and can exchange that energy between many potential donors and acceptors. We will see many examples of ATP "at work" in the cell, so be looking for them. As you see them, try to think of them as functional examples of Nature's uses for ATP that you could be expected to see in another reaction or context.
ATP structure and function
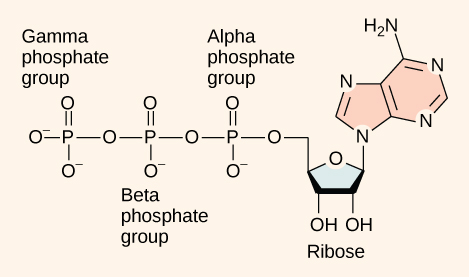
At the heart of ATP is the nucleotide called adenosine monophosphate (AMP). Like the other nucleotides, AMP is composed of a nitrogenous base (an adenine molecule) bonded to a ribose molecule and a single phosphate group. The addition of a second phosphate group to this core molecule results in the formation of adenosine diphosphate (ADP); the addition of a third phosphate group forms adenosine triphosphate (ATP).
Figure 1. ATP (adenosine triphosphate) has three phosphate groups that can be removed by hydrolysis to form ADP (adenosine diphosphate) or AMP (adenosine monophosphate).
The phosphorylation (or condensation of phosphate groups onto AMP) is an endergonic process. By contrast, the hydrolysis of one or two phosphate groups from ATP, a process called dephosphorylation, is exergonic. Why? Let's recall that the terms endergonic and exergonic refer to the sign on the difference in free energy of a reaction between the products and reactants, ΔG. In this case we are explicitly assigning direction to the reaction, either in the direction of phosphorylation or dephosphorylation of the nucleotide. In the phosphorylation reaction the reactants are the nucleotide and an inorganic phosphate while the products are a phosphorylated nucleotide and WATER. In the dephosphorylation/hydrolysis reaction, the reactants are the phosphorylated nucleotide and WATER while the products are inorganic phosphate and the nucleotide minus one phosphate.
Since Gibbs free energy is a state function, it doesn't matter how the reaction happens; you just consider the beginning and ending states. As an example, let's examine the hydrolysis of ATP. The reactants ATP and water are characterized by their atomic makeup and the kinds of bonds between the constituent atoms. Some free energy can be associated with each of the bonds and their possible configurations—likewise for the products. If we examine the reaction from the standpoint of the products and reactants and ask "how can we recombine atoms and bonds in the reactants to get the products?," we find that a phosphoanhydride bond between an oxygen and a phosphorus must be broken in the ATP, a bond between an oxygen and hydrogen must be broken in the water, a bond must be made between the OH (that came from the splitting of water) and the phosphorus (from the freed PO3-2), and a bond must be formed between the H (derived from the splitting of water) and the terminal oxygen on the phosphorylated nucleotide. It is the sum of energies associated with all of those bond rearrangements (including those directly associated with water) that makes this reaction exergonic. A similar analysis could be made with the reverse reaction.
Possible Exercise
Use the figure of ATP above and your knowledge of what a water molecule looks like to draw a figure of the reaction steps described above: breaking of the phosphoanhydride bond, breaking of the water, and formation of new bonds to form ADP and inorganic phosphate. Track the atoms in different colors if that helps.
Is there something special about the specific bonds involved in these molecules? Much is made in various texts about the types of bonds between the phosphates of ATP. Certainly, the properties of the bonds in ATP help define the molecule's free energy and reactivity. However, while it is appropriate to apply concepts like charge density and availability of resonance structures to this discussion, trotting these terms out as an "explanation" without a thorough understanding of how these factors influence the free energy of the reactants is a special kind of hand-waving that we shouldn't engage in. Most BIS2A students have not had any college chemistry and those who have are not likely to have discussed those terms in any meaningful way. So, explaining the process using the ideas above only gives a false sense of understanding, assigns some mystical quality to ATP and its "special" bonds that don't exist, and distracts from the real point: the hydrolysis reaction is exergonic because of the properties of ATP and ALSO because of the chemical properties of water and those of the reaction products. For this class, it is sufficient to know that dedicated physical chemists are still studying the process of ATP hydrolysis in solution and in the context of proteins and that they are still trying to account for the key enthalpic and entropic components of the component free energies. We'll just need to accept a certain degree of mechanistic chemical ignorance and be content with a description of gross thermodynamic properties. The latter is perfectly sufficient to have deep discussions about the relevant biology.
"High-Energy" bonds
What about the term "high-energy bonds" that we so often hear associated with ATP? If there is nothing "special" about the bonds in ATP, why do we always hear the term "high-energy bonds" associated with the molecule? The answer is deceptively simple. In biology the term "high-energy bond" is used to describe an exergonic reaction involving the hydrolysis of the bond in question that results in a "large," negative change in free energy. Remember that this change in free energy does not only have to do with the bond in question but rather the sum of all bond rearrangements in the reaction. What constitutes a large change? It is a rather arbitrary assignment usually associated with an amount of energy associated with the types of anabolic reactions we typically observe in biology. If there is something special about the bonds in ATP, it is not uniquely tied to the free energy of hydrolysis, as there are plenty of other bonds whose hydrolysis results in greater negative differences in free energy.
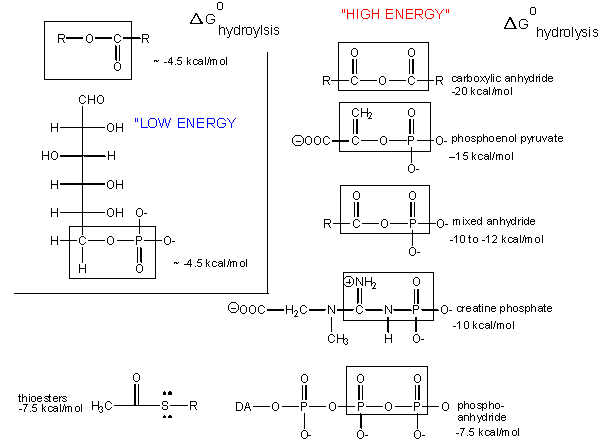
Figure 2. The free energy of hydrolysis of different types of bonds can be compared to that of the hydrolysis of ATP. Source: http://bio.libretexts.org/Core/Biochemistry/Oxidation_and_Phosphorylation/ATP_and_Oxidative_Phosphorylation/Properties_of_ATP
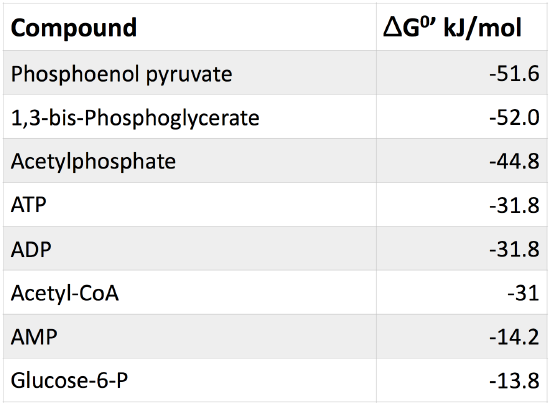
Table 1. Table of common cellular phosphorylated molecules and their respective free energies of hydrolysis.
Video on electron and electron/proton carriers
For a seven-minute YouTube video on the role of carriers in respiration click here.
External link discussing the energetics of coupling ATP hydrolysis to other reactions
The cycling of ATP pools
Estimates for the number of ATP molecules in a typical human cell range from ~3x107 (~5x10-17 moles ATP/cell) in a white blood cell to 5x109 (~9x10-15 moles ATP/cell) in an active cancer cell. While these numbers might seem large, and already amazing, consider that it is estimated that this pool of ATP turns over (becomes ADP and then back to ATP) 1.5 x per minute. Extending this analysis yields the estimate that this daily turnover amounts to roughly the equivalent of one body weight of ATP getting turned over per day. That is, if no turnover/recycling of ATP happened, it would take one body weight worth of ATP for the human body to function, hence our previous characterization of ATP as a "short-term" energy transfer device for the cell.
While the pool of ATP/ADP may be recycled, some of the energy that is transferred in the many conversions between ATP, ADP, and other biomolecules is also transferred to the environment. In order to maintain cellular energy pools, energy must transfer in from the environment as well. Where does this energy come from? The answer depends a lot on where energy is available and what mechanisms Nature has evolved to transfer energy from the environment to molecular carriers like ATP. In nearly all cases, however, the mechanism of transfer has evolved to include some form of redox chemistry.
In this and the sections that follow we are concerned with learning some critical examples of energy transfer from the environment, key types of chemistry and biological reactions involved in this process, and key biological reactions and cellular components associated with energy flow between different parts of the living system. We focus first on reactions involved in the (re)generation of ATP in the cell (not those involved in the creation of the nucleotide per se but rather those associated with the transfer of phosphates onto AMP and ADP).
Video link
For a more detailed explanation of ATP and how this molecule stores energy, take a look at this video (10 minutes) by clicking here.
How do cells generate ATP?
A variety of mechanisms have emerged over the 3.25 billion years of evolution to create ATP from ADP and AMP. The majority of these mechanisms are modifications on two themes: direct synthesis of ATP or indirect synthesis of ATP with two basic mechanisms known respectively as substrate level phosphorylation (SLP) and oxidative phosphorylation. Both mechanisms rely on biochemical reactions that transfer energy from some energy source to ADP or AMP to synthesize ATP. These topics are substantive, so they will be discussed in detail in the next few modules.