B3. Thermodynamics of Membrane Potential
- Page ID
- 5591
How can this equilibrium potential be calculated? The total thermodynamic driving force, \(ΔG_{tot}\), for an ion \(X\) moving across the membrane (from inside to outside) of transmembrane potential ΔΨ is the sum of the ΔGs arising from concentration gradients and from the electrical potential:
\[ΔG_{tot} = ΔG_{ion grad} + ΔG_{elect pot}\]
The ΔGelect pot can be determine from the simple general chemistry equation:
\[ΔG_{elect pot}= -nFE = -nFΔΨ\]
where F, the Faraday constant (the electric charge on one mol of electric charge = 23,000 cal/V.mol), n (or z) is the number of moles of charge transferred per mol of ions moving (+ 1 for Na and K ions) and ΔΨ ( or E) is the transmembrane electrical potential in volts. Hence for the movement of an ion from inside to outside the cell,
\[ΔG_{tot} = [G_{out} - G_{in}] - zFDY\]
This shows that the total driving force for ion movement consists of a chemical potential (first term) plus an electrical potential (second term). The equation can be expanded and rearranged as follows, where \(\chi_{in}\) and \(\chi_{out}\) are the concentrations of ion \(X\) inside and outside the cell, respectively:
\[ΔG_x = (ΔG_x^o + RT\ln \chi_{out}) - (ΔG_x^o + RT\ln \chi_{in}) - zFDY\]
\[ΔG_x = RT \ln \chi_{out} - RT\ln \chi_{in} - zFDY\]
\[ΔG_x = RT \left(\dfrac{\chi_{out}}{\chi_{in}}\right) - zFDY \label{1}\]
\[ΔG_x = 2.303 RT \log_{10} \left(\dfrac{\chi_{out}}{\chi_{in}}\right) - zFDY \label{2}\]
Consider \(\ce{K^{+}}\), where \(\chi_{out}/\chi_{in}\) is about 1/20 = 0.05. (The table above suggests that it is more like 1/35, but some texts use the value of 20.) What would the transmembrane potential have to be so that no driving force would exist for \(\ce{K^{+}}\) ions to move across the membrane (i.e. under equilibrium conditions)? Under these conditions, ΔGx = 0, so
\[2.303RT log(Xout/Xin ) = zFΔΨeq \label{3}\]
or
\[ΔΨeq = (2.303RT/zF) log(Xout/Xin )\label{4}\]
or
\[ΔΨeq = (0.0615/z) log(Xout/Xin) at 310K or 37oC) \label{5}\]
or
\[ΔΨeq = (0.0591/z) log(Xout/Xin ) at 298K or 25oC \label{6}\]
Equations \ref{3}-\ref{6} are versions of the Nernst equation. For K+ ions, ΔΨ is about -0.075 V or -75 mV (assuming 37oC = 310K, R = 1.98 cal/(K.mol), and z = +1). That is, since the inside is more negative than the outside, then K ions would tend to stay inside even if the chemical potential of K ions is greater on the inside. A similar calculation for Na ions, with a Xout/Xin of about 12, gives an equilibrium Na ion potential of about +55 mV. Why is the actual transmembrane potential close to the equilibrium potential of K ions and not that of Na ions? The reason has to do with the fact that the permeability of K+ ions is 100 fold greater than of Na ions. Hence it is the K ions which mostly determine the cell resting potential. (Remember glial cells have only a nongated K channel.)
In general, the ΔΨeq is determined by more than one ion (usually Na and K), so the actual ΔΨeq is between the ΔΨeq for each ion alone. A more general equation can be derived which shows that the ΔΨeq is determined not only by the concentrations but also the permeability of these ions. (In a liposome made with encapsulated KCl at a higher concentration than the outside concentration, and with both sides being electrically neutral, if no ions could flow across the membrane, no membrane potential would arise.) The equation that considers both concentration AND permeability effects is the Goldman equation and is given by:
DYeq = (RT/F) ln {[PKK+out + PNaNa+out + PClCl-in] / [PKK+in + PNaNa+in+ PClCl-out}
DYeq = 2.303 (RT/F) log {[PKK+out + PNaNa+out + PClCl-in] / [PKK+in + PNaNa+in + PClCl-out]}
DYeq (in volts) = 0.059 log {[PKK+out + PNaNa+out + PClCl-in] / [PKK+in + PNaNa+in + PClCl-out]}
DYeq (in mV) ~ 59 log {[PKK+out + PNaNa+out + PClCl-in] / [PKK+in + PNaNa+in + PClCl-out]}
where PX is the permeability of ion X. This treatment recognizes the importance of thermodynamics (chemical and electrical potentials) and kinetics (permeability coefficients). Remember this potential would not be possible if the ion gradient was not maintained by Na/K ATPase. To see the effects on the transmembrane voltage use the Goldman Equation Calculators below and plug in the following generally realistic values for concentrations and permeabilities of ions in neurons:
| ion | inside (mM) | outside (mM) | permeability coeff (cm/s) |
|---|---|---|---|
| K+ | 140 | 5 | \(1 \times 10^{-8}\) |
| Na+ | 12 | 145 | \(1 \times 10^{-10}\) |
| Cl- | 3 | 145 | \(1 \times 10^{-10}\) |
Then increase the permeability of Na ions and watch the effect on the transmembrane voltage. Reset the Na ion permeabilty and change that for chloride ion.
Goldman Equation simulator- Goldman Equation Calculator (not updated with new Java, 3/18/14)
- Applets for action potentials (not updated with new Java, 3/18/14)
Fluorophores that insert into membranes and whose flourescence changes with transmembrane potential have been developed. An example is di-4-ANEPPS, a AminoNaphthylEthenylPyridinium dye, whose structure is shown below.
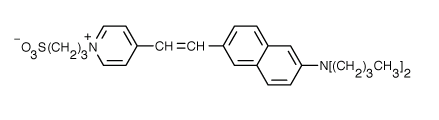
When bound to membranes, this probe has an excitation and emission maximum of 475 and 617 nm, respectively. When the membrane potential is made more negative (hyperpolarized), the fluorescence intensity (excitation 440 nm) decreases while the emission increases when excited at 530 nm.
- Use of Membrane Potential-Sensitive Fluorophores In Determination of Membrane Potential
In the 1940's, ways were developed to measure the actual transmembrane potential of cells. Varying the outside sodium and potassium concentrations would change the experimental transmembrane potential, as indicated by the Nernst equation. The experimental resting potentials of glial cells always matched the theoretical potassium equilibrium potentials, supporting the view that the transmembrane potential was associated only with open, nongated potasisum channels. This was not observed with neurons, suggesting that channels other than for potassium were open. Using radioactive tracers and potential measurements, it became clear that nerve cells were permeable not only to potassium, but also to sodium and chloride. How do these work in establishing the resting potential? Consider the simplest case when just potassium channels are present, along with an unequal distribution of other ions. Now add some sodium channels. Two forces act to drive sodium into the cell - the chemical potential since sodium is higher on the outside, and the electrical potential since the inside of the cell is negative. The equilibrium potential of a cell if it were only permeable to sodium is +55 mv, so there is a great electrical drive for sodium to enter through the nongated, open sodium pores we just added. As sodium enters, the cell starts to "depolarize" and have a more positive voltage. However, since in our example, there are many more open potassium channels, the resting potential deviates only a small amount from the potassium potential, since as the potential becomes more positive, more potassium flows out down the concentration gradient. Eventually the enhanced potassium efflux equals the sodium influx, and a new resting membrane potential of -60 mV is established, which is typical of neurons.
Now we can answer question 3 above: How is the resting electrochemical potential and the transmembrane ion distribution maintained? For a resting electrical potential to be maintained once established, the rate of sodium influx must equal the rate of potassium efflux through the open, non-gated channels in order to maintain separation of charge (and the electrical potential) across the membrane. But, if influx and efflux were allowed to continue, the actual ion gradients (chemical potential) across the membranes would collapse. This problem is solved by the Na/K ATPase. In the resting cells, the passive fluxes of sodium and potassium ions are exactly balanced by the active fluxes of these ions mediated by the Na/K ATPase. The cell is not really at equilibrium but at a steady state.
Contributors